sábado, 14 de julho de 2018
sexta-feira, 13 de julho de 2018
U.S. Wins Romanian Master of Mathematics Competition 59th International Mathematical Olympiad (IMO) which took place in Cluj-Napoca, Romania on July 3-14, 2018
WASHINGTON, DC - The U.S. team won first place for the third time in four years at the 59th International Mathematical Olympiad (IMO) which took place in Cluj-Napoca, Romania on July 3-14, 2018, with 116 countries participating. Prior to a fourth place finish in 2017, the U.S. IMO team won first place in 2015 and 2016 in the prestigious international competition. In 2018, the International Mathematical Olympiad brought together the top math students from around the world with 615 student competitors. The six U.S. team members also took home five gold medals and one silver medal for their individual high scores in the competition, known as the olympics of mathematics competitions for high school students. The first place U.S. team score was 212 out of a possible 252 points.
The teams from Russia and China took second and third place respectively in cumulative team scores. The 2018 U.S. International Mathematical Olympiad team is: Adam Ardeishar, Andrew Gu, Vincent Huang, James Lin, Michael Ren, and Mihir Singhal. Gu, Huang, and Lin are returning team members from 2017 and Lin earned a perfect score.
LINK:https://www.maa.org/news/team-usa-returns-to-first-place-in-olympics-of-high-school-math?utm_source=website&utm_medium=homepage&utm_campaign=AMC
USA---------POSITION 1
RUSSSIA---POSITION 2
CHINA------POSITION 3
BRAZIL------ POSITION 28
GERMANY----POSITION 31
FRANCE-------POSITION 33
PERU-----------POSITION 35
MEXICO-------POSITION 36
ARGENTINA-POSITION 39
COLOMBIA --POSITION 66
BOLIVIA------POSITION 81
CHILE ---------POSITION 91
PARAGUAY---POSITION 95
LINK RESULTS
http://imo-official.org/results.aspx
segunda-feira, 9 de julho de 2018
domingo, 8 de julho de 2018
59TH INTERNATIONAL MATHEMATICAL OLYMPIAD CLUJ-NAPOCA - ROMANIA, 03 - 14 JULY 2018
ABOUT IMO
The International Mathematical Olympiad (IMO) is the largest, oldest and most prestigious scientific Olympiad for high school students. The history of IMO dates back to 1959, when the first edition was held in Romania with seven countries participating: Romania, Hungary, Bulgaria, Poland, Czechoslovakia, East Germany, and USSR. Since then, the event has been held every year (except 1980) in a different country. Currently, more than 100 countries from 5 continents participate. Each country can send a team of up to six secondary students or individuals who have not entered University or the equivalent, as of the date of celebration of the Olympiad, plus one team leader, one deputy leader, and observers if desired. During the competition, contestants have to solve, individually, two contest papers on two consecutive days, with three problems each day. Each problem is worth seven points. Gold, silver, and bronze medals are awarded in the ratio of 1:2:3 according to the overall results — half of the contestants receive a medal. In order to encourage as many students as possible to solve complete problems, certificates of honorable mention are awarded to students (not receiving a medal) who obtained 7 points for at least one problem.
segunda-feira, 2 de julho de 2018
domingo, 24 de junho de 2018
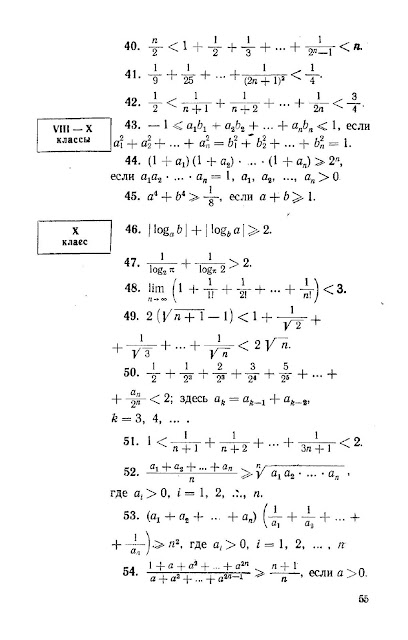
Course of Mathematical Analysis, Part 1, Vinogradov OL, Gromov - Курс математического анализа, Часть 1, Виноградов О.Л., Громов А.Л., 2009.
Course of Mathematical Analysis, Part 1, Vinogradov OL, Gromov AL, 2009
The book is the first part of the course of mathematical analysis, read at the Faculty of Mathematics and Mechanics of the St. Petersburg State University. The volume and content of the first part roughly corresponds to the material traditionally included in the first semester of a five-semester or four-semester (with a separate semester course of the theory of functions of a complex variable) course. It includes the following sections: introduction, the theory of limits and continuous functions, the differential calculus of functions of one real variable, an indefinite integral.
LINK
http://www.mediafire.com/file/f0k9igg556qjp59/vinogradov-part1.pdf
Assinar:
Postagens (Atom)